What is descriptive geometry?

Table of contents
Since the dawn of time, mankind has sought ways to accurately represent space in a comprehensible and orderly manner. Although simple drawings or diagrams have served humans since antiquity, it was not until the work of Gaspard Monge in the 18th century that these efforts were given mathematical accuracy and universality. At its core, descriptive geometry is the science of projecting spatial objects onto two-dimensional planes in a precise and unambiguous manner.
What is the basis of descriptive geometry?
The central idea of descriptive geometry is that projections onto planes can represent complex three-dimensional solids. This allows for the analysis of their structure and spatial relationships while remaining in the realm of two-dimensional drawings. This approach is of great importance in engineering and architecture, where it is often crucial to precisely plan the structure of a building or machine before proceeding to its implementation.
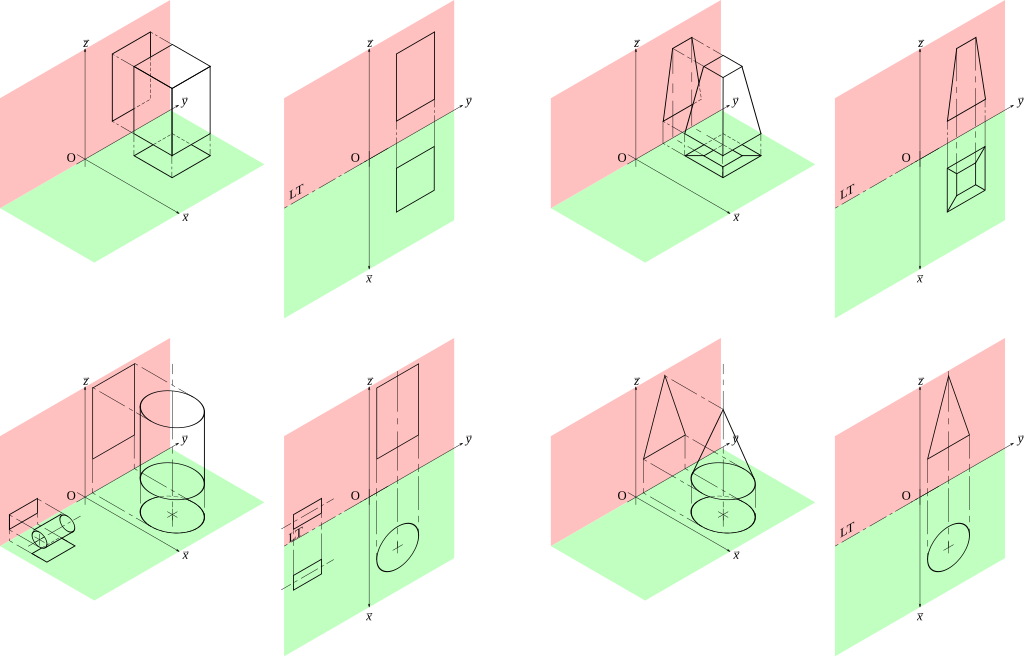
Descriptive geometry provides an easy way to understand space. Thanks to the rectangular projection – one of the basic techniques of this field – it is possible to look at a solid from different perspectives, making this method an extremely versatile tool. An image seen from above (horizontal projection), from the side ( lateral projection ), or from the front (vertical projection) captures a lot of information about an object that can be analyzed in a design context.
Its advantage is its ability to combine theoretical mathematics with practical applications. The design of buildings, bridges, or machinery is based on the ability to predict how the various elements will interact in reality. Thanks to Monge’s methods, engineers were able to plan and solve construction problems before work even began on the construction site.
Genesis and development of the discipline
Although now considered a fundamental branch of applied mathematics, descriptive geometry owes its origins to practical needs. In the 18th century, when Gaspard Monge began his career as a young military engineer, he encountered a problem requiring rigorous modeling of space in the context of designing fortifications. The construction principles of such structures required great accuracy, and they had to have bombproof walls and vaults.
At the age of 18, Monge had already developed the basics of a system that allowed complex three-dimensional objects to be represented in two dimensions. However, due to the strategic importance of this knowledge, his discoveries were considered a military secret and were not made available to the public for many years. It wasn’t until 1794, after the French Revolution, that Monge shared his knowledge, introducing draining geometry into the curriculum at the École Normale and the newly founded École Polytechnique.
The introduction of draughtsman geometry into teaching at École Polytechnique in 1795 was a landmark event. In addition to his excellent mathematical skills, Monge had a gift for communicating complex ideas in an understandable and inspiring way. Already during his first lectures, the new field aroused great interest among students and became the basis of technical science.
Monge defined draughtsman geometry as a tool with two main goals: first, to enable the precise representation of three-dimensional objects in two dimensions, second, to draw conclusions about the shapes and relationships between these objects. Thanks to these principles, the new field gained theoretical and practical dimensions, allowing it to spread rapidly.

Basic concepts of descriptive geometry
The central element of descriptive geometry is rectangular projections, which allow three-dimensional objects to be represented on a flat surface in an unambiguous way. This technique allows for creating drawings that reflect different views of an object: from above, from the front,, and from the side. Each projection shows various aspects of the structure, allowing a full understanding of its geometry.
Rectangular projections, for example, demonstrate their practicality in building design. A building’s floor plan shows the layout of rooms and the arrangement of doors and windows, while vertical projections show the height and proportions of the facade. In addition, side projections allow analysis of the depth and details of the structure, such as roof protrusions or facade details.
Drawing geometry also makes it possible to solve complex spatial problems, such as the interpenetration of solids. In architecture, the example is used to illustrate vaulted roofs, helping to determine how the various elements come together. In mechanical engineering, these techniques are used in the design of machine parts, where each component must fit perfectly with the others.
A key feature of descriptive geometry is its versatility. The projection method allows creating images of both simple geometric figures and complex shapes, such as ellipsoidal or hyperboloid surfaces. The introduction of this technique into design practice was the invaluable contribution of Gaspard Monge, who turned descriptive geometry into a tool that combines mathematical precision with aesthetics and functionality.
Thanks to descriptive geometry, it also became possible to visualize concepts previously remaining in the realm of imagination. These techniques are not only a tool for creating drawings but also a tool for spatial analysis to understand and optimize designs.
Monge’s applications and innovations
One of Gaspard Monge’s most impressive achievements was the application of the principles of descriptive geometry to the solution of practical construction problems. His research was not limited to the creation of theoretical spatial models but also included specific engineering methods, such as stereotomy – the science of cutting stone, whose application to vaulting and mortarless construction revolutionized 18th-century architecture.
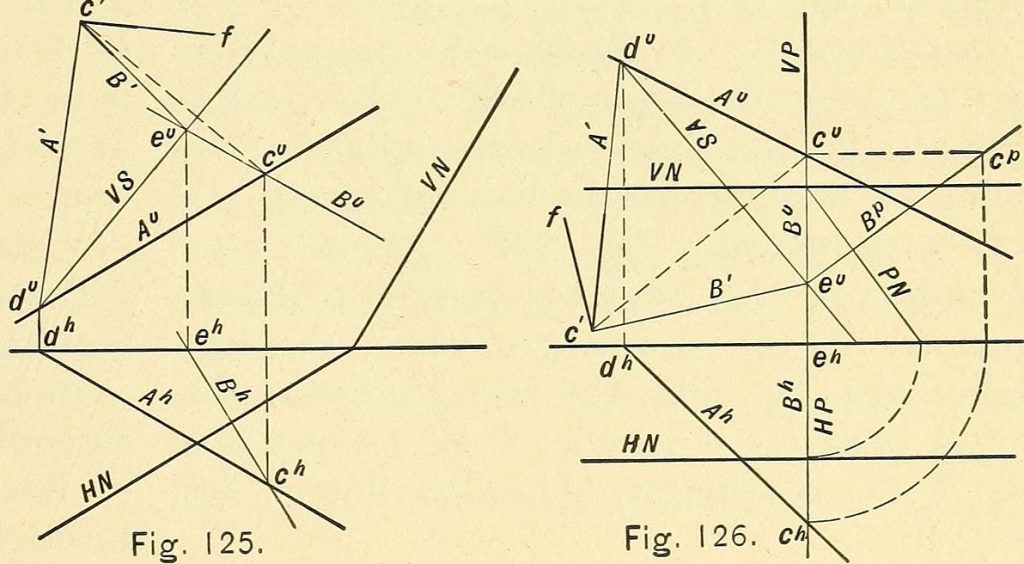
Monge noted that a key element in successful design is a proper understanding of the lines of curvature on the surface of objects. These lines, an orthogonal arrangement on the surface, provide an aesthetic emphasis on its character and, most importantly, facilitate the planning of the division of structural elements. These principles allowed stone vaults to be assembled from precisely matched elements that formed stable structures without using mortar.
Monge’s innovation was not limited to architectural structures. His methods have also been applied in mechanical engineering, where design accuracy is crucial. Rectangular projection was used in machine design, allowing for the precise definition of the shapes and proportions of individual parts and how they fit together.
Another breakthrough was the combination of descriptive geometry and aesthetics. Monge believed that construction lines should reflect the character of the surface on which they are located. With this, he introduced a new quality to architectural design, where form and functionality complement each other. Dividing lines on vaults or building facades became a decorative element, giving a harmonious appearance to the whole.
Monge showed that draughtsman geometry is not just a mathematical tool but a universal language that combines theoretical foundations with the practical needs of construction and design. As a result, his achievements remain relevant and inspire successive generations of designers.
Criticism and influence of descriptive geometry
Despite the revolutionary nature of descriptive geometry and its versatile applications, Gaspard Monge’s theory was not free of limitations and faced criticism, especially in the context of more complex structures. The first and most prominent problem was the difficulty of applying the principles of stereotomy to structures with more unusual shapes, such as diagonal bridges or vaults with non-expandable surfaces.
One example of these limitations was bridges built in Europe in the 19th century, particularly structures designed for train passage. In the case of diagonal bridges, where the angle between the road and the railroad was slight, Monge’s descriptive geometry did not always provide adequate tools for optimal force distribution. As critics pointed out, the lines of curvature that Monge proposed as a key design element did not always correspond to actual mechanical requirements, such as pressure distribution or resistance to vibration.
While fully justified, these criticisms do not diminish Monge’s importance as the founder of the basic methodology of spatial geometry. His work inspired generations of engineers who developed and adapted his methods to new technical challenges. In the second half of the 19th century, for example, design techniques evolved with the development of elasticity theory and materials science, which allowed for a better understanding of the complex mechanical behavior of structures.
Monge’s influence was also evident in the later development of architecture and engineering in the 20th century. In the modern era, structures such as concrete shells and lightweight steel structures introduced entirely new design possibilities, but at the same time were based on the basic principles of descriptive geometry. Monge’s ideas, combining theory and practice, were the foundation for developing these innovative technologies.
Monge also left a lasting mark on technical education. His approach, emphasizing practical applications of geometry, has been adopted in technical schools worldwide. His teaching methods, based on a clear presentation of the relationship between spatial solids and their projections, have become the basis for the education of engineers and architects.
In summary, the criticism faced by some aspects of Monge’s theory was a starting point for the further development of descriptive geometry rather than its denial. The impact of his work extends far beyond the times in which he lived, and his methods remain a key element of modern engineering and architecture. Monge showed that geometric thinking can be a tool for analysi and a source of innovation that changes the world.

Descriptive Geometry – Summary
Gaspard Monge’s descriptive geometry was a fundamental step toward organizing spatial thinking. It combined the precision of mathematics with the practical requirements of architecture and engineering. The introduction of rectangular projections to represent three-dimensional reality on a two-dimensional plane changed how people designed and understood structures.
Despite later criticism and challenges to more complex designs, Monge laid a foundation developed and refined over the following centuries. Descriptive geometry became a universal tool, a design language that is still taught to this day by engineers and architects alike. The idea of combining functionality with aesthetics in spatial design gained a new dimension thanks to Monge. His legacy remains evident in any work that involves spatial thinking.